Conservation of Momentum

Cut to the Chase

Momentum

Momentum is defined as the mass multiplied by the velocity. p= mv. Since the momentum depends on the velocity, it is also a vector quantity. The units for momentum are [kg.m.s^-1]

Momentum is the mass multiplied by the velocity. p=mv.

The rate of change in momentum, we explained in the section on Newton's laws of motion is another definition of the force. If we measure the momentum of a object over a small period of time, F = (mv - mu)/δt.

Impulse

Taking the time to the left-hand-side of the equation, F δt = mv - mu. The force multiplied by the time has a special name, the impulse. The impulse is the change in the momentum. Impulse is a vector with units of [kg.m.s^-1] or since [kg.ms^-2] is equivalent to a Newton [N], the units of impulse are [N.s].

Impulse is the force multiplied by time the force acts on an object. I=Ft

Consider a ball that is thrown into the air. We can make the ball go higher in two ways, firstly, we can apply a larger force, F or we can make the same force act on the ball for a longer time.

If the force acting on the object is not constant over the time for the time in which the force acts, the impulse is given by the integral of the force with respect to time.

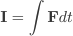
Conservation of Momentum

The conservation is an important principle in physics that allows us to determine what happens in collisions or explosions. If a body A of mass m_A and velocity u_A collides with another body B of mass m_B and velocity v_B moving in the same direction. If A exerts a force F to the right on B for a time t then by Newton's third law, body B will exert an equal and opposite force to the left on A also for a time t but to the left. Thus the bodies receive equal but opposite impulse Ft. The changes of momentum must be equal and opposite. Therefore, the total momentum change is zero. In other words, the total momentum of A and B together remains constant. In mathematical terms:

This is the general case. For motion in 1-dimension, we can consider the momenta in one direction only which allows us to drop the vector notation.

It is important to release that the momentum is conserved only when there are no external agent acting on the system that could add momentum to the system. They must be included in the system before the conservation of momentum can be applied.

A good example of this is a ball that is dropped from a height. If we are considering the ball alone, then the momentum is m_bv_b before the ball collides and -m_bv_b when the ball hits the Earth. The change in momentum is 2m_bv_b not zero. It appears to gain momentum from nowhere. However, we need to consider the change in momentum of the Earth. It moves with a velocity v_E after the collision. The conservation of momentum, m_bv_b + m_E.0 = m_b(-v)_b+m_Ev_E. The Earth has to gain the same momentum as the ball but because the mass of the Earth is so large, the movement is unmeasurable.

Elastic and Inelastic Collisions

Momentum is always conserved in any closed system, however kinetic energy is not always conserved and this brings us neatly to the subject of elastic and inelastic collisions.

• An elastic collision is a collision where the both the momentum and the kinetic energy are conserved.
• In an inelastic collision the momentum is conserved but the kinetic energy is not. In fact the kinetic energy will be lower after the collision because some energy has been used in creating sound, deformation and heat. The reality is that most collisions are inelastic to some extent. However, collisions between snooker balls come quite close to being elastic collisions.

Collisions in 1-d.

In the flash animation above, the conservation of momentum is illustrated by the collision between two trolleys A and B. Initially, trolley B is stationary and trolley has a velocity v_A also the masses m_A and m_B are the same.

Applying the conservation of momentum gives, m_A u_A+ 0 = m_Av_A + m_Bv_B. In the event that the two masses are the same, then mass m_B will move off at the same speed and mass m_A will stop.

To calculate what happens when the masses are different we need to use a second equation. u_A = v_A+v_B. This states that the incoming velocity is equal to the sum of the final velocities. Using this equation we obtain a general solutions for v_A and v_B.

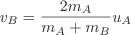
When both masses are allowed to move, their final velocities are given by:

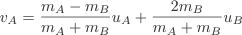
Limiting Cases

M_A>>M_B

In the limiting cases where a large mass collides with a much smaller mass, the final velocity of the small mass has a limiting value of twice the velocity of the large mass. This can be seen by looking at equation (3) since m_A+m_B ≈ m_A then v_b=2 (m_A/m_A) u_A = 2u_A.

M_A<<M_B

In the case where a small mass collides with a much larger mass, the large mass hardly moves and the small mass rebounds with a velocity similar to its entry velocity. Looking at equation (4) this can be seen m_A-m_B~-m_B and m_A+m_B~m_B therefore, v_A~-1 u_A.

Totally inelastic Collisions

So far we have considered the elastic collisions where both the kinetic energy and momentum are conserved. Most collisions are inelastic. How do we deal with those? We can measure the how much of the energy remains as kinetic energy after the collision. We take the ratio of the outgoing kinetic energy to the incoming kinetic energy. This is known as the coefficient of restitution.

e=kinetic energy after collision/kinetic energy before collision.

Explosions

In an explosion, the inital momentum is zero, after the explosion the outgoing pieces move out in opposite directions so the momentum is conserved. The idea of an explosion is replicated somewhat less dramatical with two trolleys A and B with masses m_A and m_B respectively, which are initially held together. Momentum is given to the trolleys by a compressed spring. When the spring is released the momentum is given to the trolleys which move apart in opposite directions.

Applying the conservation of momentum, the momentum before the explosion is 0 since neither trolley is moving and after the explosion the momentum is m_Av_A-m_Bv_B. Therefore, the change in momentum is m_Av_A-m_B-0 or m_Av_A=m_Bv_B.